MULTIPLICATION SHORT CUT METHODS UP 100 X 100 FOR ALL EXAMS
Multiplication Short cut methods use full for all exams. All the best
MULTIPLICATION UP TO 20X20 IN YOUR HEAD
- Take 15 x 13 for an example.
- Always place the larger number of the two on top in your mind.
- Then draw the shape of Africa mentally so it covers the 15 and the 3 from the 13 below. Those covered numbers are all you need.
- First add 15 + 3 = 18
- Add a zero behind it (or multiply by 10) to get 180.
- Multiply the covered lower 3 x the single digit above it the "5" (3x5= 15)
- Add 180 + 15 = 195.
15 X 13 = 195
MULTIPLICATION SHORT CUT METHODS UP 100 X 100
To multiply two numbers (of two or more digits), split each number into two parts. If the first number is a1 + b1 and the second number is a2 + b2, then the product of the two numbers is:
(a1 x a2) + (a1 x b2 + b1 x a2) + (b1 x b2)
Long Multiplication shortcut technique
The solution comprises three parts (as shown by the boxes and arrows above): the head, the middle, and the tail.
1. The digits on the right are multiplied vertically to get the tail part: b1 x b2 (excess carried over)
2. All digits are multipled crosswise and added together to get the middle part: a1 x b2 + b1 x a2 (excess carried over)
3. The digits on the left are multiplied vertically to get the head part: a1 x a2
Here is a simple example to illustrate this technique.
- 23 x 41 = 943
Long Multiplication technique example
The steps are:
1. 3 x 1 = 3
2. 2 x 1 + 3 x 4 = 14, put down 4 and carry over 1
3. 2 x 4 = 8, plus the 1 carried over, is 9
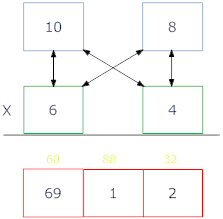
The speed gain using this technique (over the conventional method of multi-line long multiplication) becomes more apparent when handling larger numbers. Here is another example involving excess carryover at each stage.
- 108 x 64 = 6912
Long Multiplication technique example
The steps are:
1. 8 x 4 = 32, put down 2 and carry over 3
2. 10 x 4 + 8 x 6 = 88, plus the 3 carried over, is 91; put down 1 and carry over 9
3. 10 x 6 = 60, plus the 9 carried over, is 69
MULTIPLICATION UP TO 20X20 IN YOUR HEAD MULTIPLICATION SHORT CUT METHODS UP 100 X 100 Easy Multiplication Shortcuts for calculation & All Bank Exams MORE MULTIPLICATION SHORT CUTS FOR MATHS or MATHEMATICS MULTIPLICATION SHORT CUT METHODS UP 100 X 100 FOR ALL EXAMS AND CHILDREN
